January 16th 2012
CH-112
Chapter 12
Solids & Modern Materials
Metallic solids: held together by a delocalized sea of collectively shared valence electrons. Because they are held together by this type of bond, they are electrically conductive, relatively strong and not brittle.
Ionic solids: held together by the mutual attraction between cations and anions.
Covalent-network solids: held together by an extended network of covalent bonds. Results in extremely hard materials (ie: diamond) and the unique properties of semi-conductors.
Molecular solids: held together by intermolecular forces: dispersion forces, dipole-dipole interactions, and hydrogen bonds. Due to the relative weakness of these forces, molecular solids tend to have low boiling points.
Polymers: contain long chains of atoms, atoms within a given chain are connected by covalent bonds and adjacent chains are held to one another largely by weaker intermolecular forces. Polymers are stonger and have higher melting points than molecular solids and are more flexible than other bonded types of solids.
Nanomaterials: solids in which the dimensions of individual crystals have been reduced to 1-100nm. The decrease in dimensions of crystals in conventional materials changes their properties.
The structures of solids tend to have patterns repeating in three dimensions, such as the way bricks are stacked to create a wall.
Crystalline solids: solids in which atoms are arranged in an orderly repeating pattern. They typically have flat surfaces (faces) that make definite angles with one another and have highly regular shapes.
Amorphous solids (from Greek "without form"): lack the order found in crystalline solids and are similar in structure to liquids but the molecules, atoms, and/or ions lack freedom of motion. They do not have well defined shapes or faces.
Unit cell: the repeating unit in a crystalline solid made up of a unique arrangement of atoms that embodies the structure of the solid.
The structure of a crystal can be build by stacking a unit cell over and over in all three dimensions. Therefore, the structure of a crystalline solid is defined by
1. the size and shape of the unit cell
2. the locations of atoms within the unit cell
Crystal lattice: the geometrical pattern of points on which unit cells are arranged. The abstract scaffold of the crystal structure. Crysal lattices consist of lattice points and lattice vectors a and b.
Beginning from any lattice point, it is possible to move to any other lattice point by adding together whole-number multiples of the two lattice vectors.
In two dimensions, unit cells must tile in such a way that they completely cover the area of the lattice without any gaps. In three dimensions, they must stack to fill all space.

Two-dimensional lattice
Unit cells can take only one of the four shapes (The most general being oblique):
Oblique
Square
Rectangular
Hexagonal
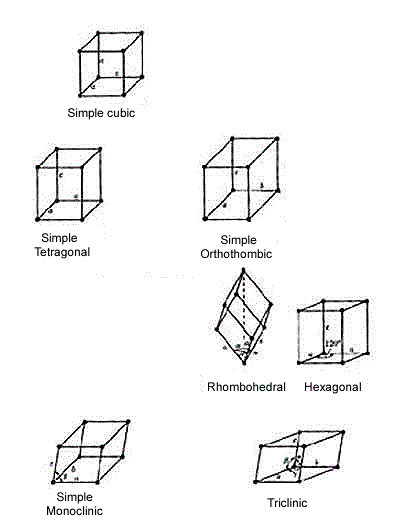
Three-dimensional crystal lattices
Three-dimensional crystal lattices are defined by three lattice vectors a, b, and c. There are seven possible shapes for three-dimensional unit cells: Cubic, Tetragonal, Orthorhomic, Rhombohedral, Hexagonal, Monoclinic, and Triclinic. These structures, with a lattic point at each corner of a unit cell, are called primitive lattices. Face centered lattices are created by placing additional lattice points in specific locations in the unit cell.
Body-centered cubic lattice: has one point at the center of the unit cell in addition to the lattice points at the eight corners.
Face-centered cubic lattice: has one lattice point at the center of each of the six faces of the unit cell in addition to the lattice points at the eight corners.
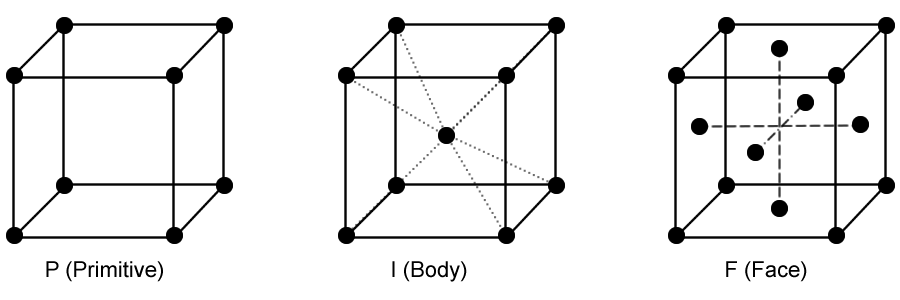
Primitive, Body, and Face lattices
The lattice alone does not define a crystal structure, this must be done by associating an atom or group of atoms with each lattice point.
In most crystals, a group of atoms is associated with each lattice point, rather than atoms being exacly coincident with lattice points. This group of atoms is called a "motif".
Graphene is a crystal structure in which no atoms lie on lattice points. Consider the following:
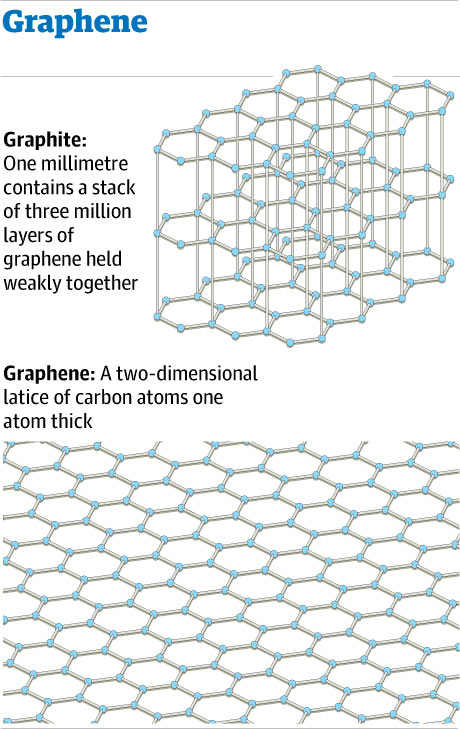
Imagine a hexagonal lattice structure over the sheet of graphene encompassing two atoms in each unit. The illustration also shows that bonds can be formed between atoms in neighboring unit cells.
Apparently, upon arriving in my first class, the email my professor sent was wrong and we started with chapter 13 rather than 12. So as cool as all this is, I am not going to finish the summary. It's been real, lattices! On to properties of solutions........